Numbers & Counting | Arithmetic | Fractions & Decimals | Prealgebra | Site Map

Rational Numbers

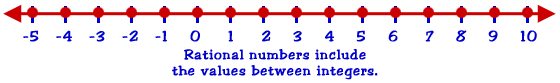
Rational numbers bring the fractions of whole numbers into our study of math. So far, we've covered whole numbers and integers. Those values are complete numbers. You could also think of them as complete objects. As we all know, sometimes you have part of an object. Maybe you have a half or a quarter. These values are between the whole values. So, when you look at a number line, almost all of the possible values are considered rational numbers. It's not just about the points where you find integers.

Rational Numbers: 1, 2, 500, -250, -36, 1/2, 1/3, -1/4, 2 2/3, -150 5/13


Rational numbers include natural numbers, whole numbers, and integers. They can all be written as fractions. Sixteen is natural, whole, and an integer. Since it can also be written as the ratio 16:1 or the fraction 16/1, it is also a rational number.

It's easy to look at a fraction and say it's a rational number, but math has its rules. The term rational number is based on the idea of ratios (1:2). As you are starting to learn, ratios can also be written as fractions (1/2).

Look at the decimal 0.5. You can get 0.5 with the division problem 1 divided by 2 (1 ÷ 2). Another way to write that division problem is 1/2. Since the 0.5 can be expressed (written as) as the fraction 1/2, 0.5 is a rational number. That 0.5 is also called a terminating decimal.

What about the decimal 0.66 . This is a repeating decimal that will never end. It's just sixes forever. Is it a rational number? Yes. You can get the value with the division problem 2 divided by 3 (2 ÷ 3). Another way to write that division problem is 2/3. Since the 0.66 can be expressed as the fraction 2/3, it is a rational number.

0.66666666666666666666666666666... 3 ) 2.00000000000000000000000000000... - 18                                                               20                                                           - 18                                                             20                                                         - 18                                                           20                                                       - 18                                                         20                                                     - 18                                                       20                                                   This will just go on forever.

Remember that the set of integers includes all of the whole numbers and their negative values. It also includes 0. You can use that 0 in a rational number if it is in the numerator (on top). However, when working with real numbers, you cannot divide by zero. You cannot have rational numbers with a 0 in the denominator. Mathematicians say that anything divided by 0 is an undefined value.

So let's look at an example. We'll pick two integers: 18 and 31. If we want to find a rational number that uses these two values, the easy one is 18/31. Don't forget that you could also make the rational number 31/18. When you learn more about fractions, you'll be able to see 31/18 as the mixed number 1 13/18. That mixed number is also a rational number, because it is a value between two whole numbers.

One more time:
• Two Integers: 5, 12
• Two possible rational numbers: 5/12 and 12/5

In division terms:
• Five divided by twelve.
• Twelve divided by five.

Both of these numbers are rational because they are found between the integer values on the number line.

5 ÷ 12 = 0.4166 (found on the number line between the integers 0 and 1)

12 ÷ 5 = 2r2 = 2.4 (found on the number line between the integers 2 and 3)

A quick note. Sometimes you get a repeating decimal when you divide two integers. You might see one third written as 0.3. That line above the three is called a vinculum. In math, it means the numbers keep repeating that way forever. Try to do the division yourself. 1÷3 gives you a never-ending solution. That's why mathematicians use the bar over the numbers. You don't need to remember the name of the bar, just remember that the bar means, "This number repeats forever."

Related Links